7. Gases
The Ideal Gas Law
7. Gases
The Ideal Gas Law - Video Tutorials & Practice Problems

Get help from an AI Tutor
Ask a question to get started.
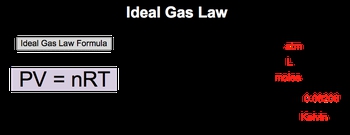
The Ideal Gas Law formula relates the behavior of gases under varying conditions of pressure, volume, moles and temperature.
The Ideal Gas Law
1
concept
The Ideal Gas Law
Video duration:
1mPlay a video:
2
example
The Ideal Gas Law Example 1
Video duration:
2mPlay a video:
3
concept
The Ideal Gas Law
Video duration:
1mPlay a video:
4
example
The Ideal Gas Law Example 2
Video duration:
2mPlay a video:
5
Problem
ProblemHow many grams of carbon dioxide, CO2, are present in a 0.150 L flask recorded at 525 mmHg and 32 ºC?
a) 1.77 g
b) 0.93 g
c) 0.66 g
d) 0.18 g
e) 0.052 g
A
1.77 g
B
0.93 g
C
0.66 g
D
0.18 g
E
0.052 g
6
Problem
ProblemHow many liters of HNO3 gas, measured at 28.0 ºC and 780 torr, are required to prepare 2.30 L of 4.15 M solution of nitric acid?
A
56 L
B
62 L
C
189 L
D
230 L
E
262 L
7
Problem
ProblemWhen 0.670 g argon is added to a 500 cm3 container with a sample of oxygen gas, the total pressure of the gases is found to be 1.52 atm at a temperature of 340 K. What is the mass of the oxygen gas in the bulb?
A
0.266 g
B
0.335 g
C
0.621 g
D
0.715 g
E
1.72 g
Do you want more practice?
We have more practice problems on The Ideal Gas Law
Additional resources for The Ideal Gas Law
PRACTICE PROBLEMS AND ACTIVITIES (57)
- At 273 K and 1 atm pressure, 1 mol of an ideal gas occupies 22.4 L. (Section 10.4) (b) Looking at Figure 18.1,...
- Many laboratory gases are sold in steel cylinders with a volume of 43.8 L. What is the mass in grams of argon ...
- Air pollution in the Mexico City metropolitan area is among the worst in the world. The concentration of ozone...
- Complete the following table for an ideal gas: P V n T 101.33 kPa ? L 3.333 mol 300 K
- (b) The adult blue whale has a lung capacity of 5.0 * 103 L. Calculate the mass of air (assume an average mola...
- (a) Calculate the number of molecules in a deep breath of air whose volume is 2.25 L at body temperature, 37 °...
- What volume is occupied by 0.118 mol of helium gas at a pressure of 0.97 atm and a temperature of 305 K?
- (b) Carbon dioxide makes up approximately 0.04% of Earth's atmosphere. If you collect a 2.0-L sample from the ...
- What volume is occupied by 12.5 g of argon gas at a pressure of 1.05 atm and a temperature of 322 K? Would the...
- (a) If the pressure exerted by ozone, O3, in the stratosphere is 304 Pa and the temperature is 250 K, how many...
- A scuba diver's tank contains 2.50 kg of O2 compressed into a volume of 11.0 L. (b) What volume would this oxy...
- An aerosol spray can with a volume of 125 mL contains 1.30 g of propane gas (C3H8) as a propellant. (c) The ca...
- An aerosol spray can with a volume of 125 mL contains 1.30 g of propane gas (C3H8) as a propellant. (a) If the...
- What is the pressure in a 15.0-L cylinder filled with 32.7 g of oxygen gas at a temperature of 302 K?
- A 50.0 g sample of solid CO2 (dry ice) is added at -100 °C to an evacuated (all of the gas removed) container ...
- A 334-mL cylinder for use in chemistry lectures contains 5.225 g of helium at 23 °C. How many grams of helium ...
- What is the temperature of 0.52 mol of gas at a pressure of 1.3 atm and a volume of 11.8 L?
- In an experiment reported in the scientific literature, male cockroaches were made to run at different speeds ...
- A piece of dry ice (solid carbon dioxide) with a mass of 28.8 g sublimes (converts from solid to gas) into a l...
- If 15.0 g of CO2 gas has a volume of 0.30 L at 300 K, what is its pressure in millimeters of mercury?
- If 2.00 g of N2 gas has a volume of 0.40 L and a pressure of 6.0 atm, what is its Kelvin temperature?
- Dry ice (solid CO2) has occasionally been used as an 'explosive' in mining. A hole is drilled, dry ice and a s...
- The reaction of sodium peroxide 1Na2O22 with CO2 is used in space vehicles to remove CO2 from the air and gene...
- A typical high-pressure tire on a bicycle might have a volume of 365 mL and a pressure of 7.80 atm at 25 °C. S...
- As shown in Table 15.2, the equilibrium constant for the reaction N21g2 + 3 H21g2 Δ 2 NH31g2 is Kp = 4.34 * 10...
- The vapor pressure of water at 25 °C is 23.76 torr. If 1.25 g of water is enclosed in a 1.5-L container, will ...
- The vapor pressure of CCl3F at 300 K is 856 torr. If 11.5 g of CCl3F is enclosed in a 1.0-L container, will an...
- Natural gas is a mixture of hydrocarbons, primarily methane 1CH42 and ethane 1C2H62. A typical mixture might h...
- Air conditioners not only cool air, but dry it as well. A room in a home measures 6.0 m * 10.0 m * 2.2 m. If t...
- A sealed flask contains 0.55 g of water at 28 °C. The vapor pressure of water at this temperature is 28.35 mm...
- A 2.85-g sample of an unknown chlorofluorocarbon decomposes and produces 564 mL of chlorine gas at a pressure ...
- The relative humidity of air equals the ratio of the par- tial pressure of water in the air to the equilibrium...
- Carbon dioxide, which is recognized as the major contributor to global warming as a 'greenhouse gas,' is forme...
- Nickel carbonyl, Ni1CO24, is one of the most toxic substances known. The present maximum allowable concentrati...
- Olympic cyclists fill their tires with helium to make them lighter. Calculate the mass of air in an air-filled...
- Olympic cyclists fill their tires with helium to make them lighter. Calculate the mass of air in an air-filled...
- Olympic cyclists fill their tires with helium to make them lighter. Calculate the mass of air in an air-filled...
- The reaction NO1g2 + NO21g2 ∆ N2O31g2 takes place in the atmosphere with Kc = 13 at 298 K. A gas mixture is pr...
- The radius of a xenon atom is 1.3 * 10 - 8 cm. A 100-mL flask is filled with Xe at a pressure of 1.0 atm and a...
- A driver with a nearly empty fuel tank may say she is 'running on fumes.' If a 15.0-gallon automobile gas tan...
- Assume that you take a flask, evacuate it to remove all the air, and find its mass to be 478.1 g. You then fil...
- The apparatus shown consists of three temperature-jacketed 1.000-L bulbs connected by stopcocks. Bulb A contai...
- When solid mercury(I) carbonate, Hg2CO3, is added to nitric acid, HNO3, a reaction occurs to give mercury(II) ...
- What is the temperature of 0.750 mol of a gas stored in a 6,850 mL cylinder at 2.21 atm?
- What is the temperature of 0.80 mol of a gas stored in a 275 mL cylinder at 175 kPa?
- What pressure will 14.0 g of CO exert in a 3.5 L container at 75°C?
- Would the volume be different if the gas was argon (under the same conditions)?
- What is the percent chlorine (by mass) in the unknown chlorofluorocarbon?
- What pressure will 14.0 g of CO exert in a 3.5 L container at 75°C?
- The volume of a sample of gas (2.49 g) was 752 mL at 1.98 atm and 62 °C. The gas is ________.
- What quantity in moles of chlorine gas at 120.0°C and 33.3 atm would occupy a vessel of 12.0 L?
- What mass of NO2 is contained in a 13.0 L tank at 4.58 atm and 385 K?
- The pressure of a sample of CH4 gas (6.022 g) in a 30.0 L vessel at 402 K is ________ atm.
- What mass of NO2 is contained in a 13.0 L tank at 4.58 atm and 385 K?
- What quantity in moles of chlorine gas at 120.0 °C and 33.3 atm would occupy a vessel of 12.0 L?
- What pressure (in atm) will 0.44 moles of CO2 exert in a 2.6 L container at 25°C?
- Determine the number of moles of air present in 1.35 L at 750 torr and 17.0°C.

